Solving Rational Equations
To solve a rational equation, such as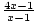 
+1 - 
= 
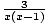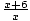, rewrite all the terms of the
equation as fractions with a common denominator. For example, 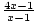+1 - 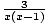= 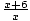can be rewritten
as:
×
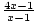+1×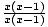
- ×
= ×
+ 
- = 
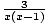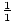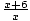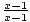
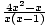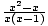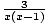Next, eliminate the denominator:
4x2 - x + x2 - x - 3 = x2 + 5x - 6
Solve the equation:
5x2 -2x - 3 = x2 + 5x - 6
4x2 - 7x + 3 = 0
(4x - 3)(x - 1) = 0
x =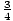 
, 1
Since we cannot divide by zero, we must check to see if any of the
x-values yield 0 in the denominator. If an x-value produces 0
in the denominator, it is not a solution. x = 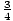does
not produce 0 in any of the denominators, but x = 1 does produce 0 in
one of the denominators. Thus, x = 1 is not a solution. The solution
set is .
Remember to check all your solutions. If a number yields zero in
any of the denominators, it is not a solution.
payment page
