sparknotes
Algebra II: Polynomials
Terms
Conjugate Zeros Theorem
-
If
P(x)
is a polynomial with real coefficients, and if
a + bi
is a zero of
P
, then
a - bi
is a zero of
P
.
Factor Theorem
-
If
P(x)
is a polynomial and
P(a) = 0
, then
x - a
is a factor of
P(x)
.
In other words, if the remainder when
P(x)
is divided by
x - a
is 0, then
x - a
is a factor of
P(x)
.
Fundamental Theorem of Algebra
-
Every polynomial function of positive degree with complex coefficients has at
least one complex zero.
Corollary. Every polynomial function of positive degree n has exactly n complex zeros (counting multiplicities).
Corollary. Every polynomial function of positive degree n has exactly n complex zeros (counting multiplicities).
Multiplicity
-
A function with
n
identical roots is said to have a zero of multiplicity
n
.
Nested Form
-
The form of a polynomial
P(x) = (((((a)x + b)x + c)x + d )x + ... )
.
Rational Zeros Theorem
-
If
P(x)
is a polynomial with integer coefficients and if
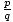is a
zero of
P(x)
(if
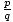P() = 0
), then
p
is a factor of the constant
term of
P(x)
and
q
is a factor of the leading
coefficient of
P(x)
.
is a
zero of
P(x)
(if
P() = 0
), then
p
is a factor of the constant
term of
P(x)
and
q
is a factor of the leading
coefficient of
P(x)
.
Remainder Theorem
-
When a polynomial
P(x)
is divided by
x - a
, the remainder is equal to
P(a)
.
Root
-
A number that, when plugged in for the variable, sets a function equal to zero.
Also called a zero.
Synthetic Division
-
A process by which a polynomial is divided by a binomial, in which the
coefficients of the polynomial are placed in a row and multiplied by and added
to the constant divisor as in nested form.
Zero
-
A number that, when plugged in for the variable, sets a function equal to zero.
Also called a root.
Become a fan on Facebook
Follow us on Twitter
